Sacred geometry deduces that certain geometrical shapes and proportions contain sacred meaning. The application of sacred geometry can be found in many civilizations around the world.

The Cambridge English Dictionary defines geometry as “the area of mathematics relating to the study of space and the relationships between points, lines, curves, and surfaces”. This definition fits nicely with the academic study of geometry, which is based on rationalism. It has been argued, however, that there is an opposite but complementary side to this field of study. According to this view, geometry has an intuitive side to it, and that certain geometrical shapes and proportion contain sacred meaning. This belief may be considered to be the basis of sacred geometry. The concept and application of sacred geometry can be found in many civilizations around the world.

Ancient Geometry: The Development of Geometry in Different Cultures

It is commonly believed that geometry began as a practical subject, and came into being as a result of everyday concerns. The earliest practitioners of geometry developed a set of rules to calculate lengths, areas, and volumes. Many of these, however, were crude approximations, and were based on trial and error. According to Herodotus, geometry was established by the ancient Egyptians . This is supported by written evidence from Egypt itself. Nevertheless, the ancient Mesopotamians are also known to have practiced geometry, as did the ancient Chinese and Indians.

Euclid is considered the father of geometry. Elements, a fragment of which is shown here, is one of the most influential works in the history of mathematics.

Around the 6th century BC, the Greeks got themselves involved in geometry, transforming it from a practical subject into an abstract one based on generalizations. It is also from the Greeks that this branch of mathematics obtained its name, as it is a combination of two Greek words, geo (earth) and metron (measure). Although there were many Greek and Roman thinkers who contributed to the subject, none had a greater impact than Euclid, who is often considered to be the father of geometry. Euclid lived in Alexandria between the 4th and 3rd centuries BC, and is best-known for his Elements. This treatise is considered to be one of the most influential works in the history of mathematics. Although commonly thought to contain only geometry, Euclid’s Elements also dealt with other areas of mathematics, i.e. elementary number theory and incommensurable lines.

Euclid’s Elements is a good example of the rational and academic approach to the study of geometry. As mentioned earlier, there were other thinkers in the classical world who contributed to the study of geometry, some of whom took a different approach to this subject. These thinkers saw symbolic and sacred meanings in geometry, and therefore their field of study may be referred to as sacred geometry. A group of these thinkers were the Pythagoreans, whose school of philosophy was founded by Pythagoras of Samos .

Pythagorean Theorem: The World’s Most Beautiful Mathematical Equation

Today, Pythagoras is best-known for the Pythagorean Theorem (known also as Pythagoras’ Theorem), which states that “the sum of the squares on the legs of a right triangle is equal to the square on the hypotenuse (the side opposite the right angle).” Although named after the Greek philosopher, the theorem is actually much older. For instance, several Babylonian clay tablets dating to between 1900 and 1600 BC show some knowledge of the theorem, and is also mentioned in the Indian Shulba Sutras, written between 800 and 400 BC. Nevertheless, the association of this theorem with the Pythagoreans is somewhat apt, as they seem to have been particularly interested in triangles.

Pythagoreans celebrating the sunrise, in an 1869 painting by Fyodor Bronnikov.

It is of little wonder then that the Pythagoreans came up with a triangle-based symbol in sacred geometry known as the tetractys, or the tetractys of the decad ( tetractys meaning “four”, and decad meaning “ten”). This symbol consists of ten dots arranged in four rows, thus forming an equilateral triangle. The tetractys is full of symbolic meaning. For example, each row of dots is supposed to contain a hidden meaning. The first row, which has a single dot, is said to represent the active principle, or divine power behind all creation; the second, the passive principle, or matter; the third, the world proceeding from the union of the active and passive principles; and the fourth, the four liberal arts and sciences that complete the world.

Naturally, the tetractys was adopted by the Pythagoreans as their symbol. In Raphael’s School of Athens, Pythagoras is depicted beside Archimedes, who is shown holding a tablet. The top of the tablet displays Archimedes’ Principle, whilst its bottom the tetractys, a nod to the Pythagoreans. The tetractys, however, was not a purely Pythagorean symbol, and that it had an influence on the sacred geometry of other cultures as well. It has been pointed out, for example, that the four letters of the Tetragrammaton (the biblical name of the God of Israel) may be arranged as a tetractys.

The Symbolic Value of Shapes

The Pythagoreans, of course, were not the only ones interested in triangles. For instance, triangular windows are a common sight on Christian churches. In this context, however, the triangle is taken to represent the Holy Trinity. As another example, triangles also possess symbolic value in the New Age movement. An upward-pointing triangle, for instance, may represent male energy, and the ascent into the spiritual realm, whereas a down-pointing one female energy, and the descent into the physical world. A combination of these two triangles, which creates a hexagram, may be interpreted as the achievement of harmony.

15th century manuscript showing Western and Arab thinkers practicing geometry.

Apart from the triangle, many other shapes have been imbued with symbolic meaning, making them part of sacred geometry. Like the triangle, these shapes may have different meanings attached to them, depending on the contexts in which they were produced. The circle, for example, is found in various cultures around the world, and therefore contains multiple meanings. The Pythagoreans, for instance, perceived the circle as a symbol of unity, oneness, and indivisibility. For Zen Buddhists, the circle is a symbol of enlightenment, whilst the Chinese regarded this shape as a symbol of the heavens. In addition, the circle is almost universally used as a symbol for the Sun. In modern astronomy, this heavenly body is represented as a circle with a dot in the middle.

The circle may be repeated in order to create new symbols. One of these, for instance, is the vesica piscis (literally meaning “bladder of a fish”), which is formed by intersecting two circles with the same radius. The two circles intersect in such a way that the center of each circle touches the perimeter of the other. The intersection of two circles in the vesica piscis is said to represent a mutual understanding, a shared vision, or common ground between two equal individuals. The vesica piscis in turn may be repeated in order to produce more symbols.

Six vesica piscis arranged radially around a central circle, or six circles fit around a seventh, all of which are of the same radius, produces a six-petalled rosette, known also as the Seed of Life. Since this symbol contains seven circles, it has been interpreted to represent creation. By repeating the six-petalled rosette, another symbol, the Flower of Life, may be produced. The Flower of Life is an ancient symbol, and is depicted at various sacred sites around the world. For some, this symbol represents the divine, mathematical order of the Universe.

Many claim that the façade of the Parthenon, part of the Acropolis in Athens, was designed using the golden ratio.

Searching for the Golden Ratio

Sacred geometry also involves geometric proportions. One of the best-known of these is the golden ratio, which is known by many other names, including phi, the divine proportion, and the golden mean. The golden ratio may be defined as follows:

“the ratio of a line segment cut into two pieces of different lengths such that the ratio of the whole segment to that of the longer segment is equal to the ratio of the longer segment to the shorter segment.”

The nautilus shell is a popular example of a golden ratio in nature.

In mathematics, it is an irrational number represented by the Greek letter φ (phi). Its value is approximately 1.618, derived from the quadratic equation  . Although this ratio was first described by Euclid, it was only referred to as ‘golden’ much later on, i.e. in 1835, when it was designated as such by the German mathematician Martin Ohm. Incidentally, it was also during the 19th century that the ratio was given the Greek letter φ to represent it. The letter was assigned by an American mathematician, Mark Barr. 

Apparently, the ancient Greeks found that the golden ratio provided “the most aesthetically pleasing proportion of sides of a rectangle,” and are alleged to have applied it in their architecture. It has been claimed, for instance, that the façade of the Parthenon in Athens was designed using the golden ratio. This has been disputed, as it has been pointed out, for instance, that the claim is not supported by actual measurements, and that the Parthenon was built about a century before Euclid’s birth.

Leonardo da Vinvi is alleged to have used the golden ratio widely in his works.

The golden ratio only attained its notorious reputation during the Renaissance, long after it was first described by Euclid. In 1509, the Italian mathematician Luca Pacioli wrote a book called De divina proportione (which translates as “The Divine Proportion”), which explored the concept of the golden ratio. Additionally, the book was illustrated by Leonardo da Vinci , who is alleged to have used the golden ratio widely in his works. The polymath’s Vitruvian Man , for example, is often claimed to have been drawn according to the golden ratio, though it has also been pointed out that there is no concrete proof to support this claim. It has also been asserted that the golden ratio exists in nature, though this is not entirely true either. A popular example is the growth of the nautilus shell, in which it is claimed that the golden ratio can be seen. It has, however, been pointed out that the shell grows in a “spiral that turns by a constant angle along its entire length”, and that a constant angle does not equal to the golden ratio.

The golden ratio is discernible in artworks such as Leonardo’s Mona Lisa.

In any event, the golden ratio is a significant concept in sacred geometry, and it is claimed to appear in both nature and the works of man. The Great Pyramid, Mozart’s musical compositions, and the growth of plants are all said to follow the golden ratio. As a result, this ratio has been imbued with symbolic value. For instance, it has been said that the golden ratio “symbolically links each new generation to its ancestors, preserving the continuity of relationship as the means for retracing its lineage.”

Platonic Solids and Plato’s Theory of the Universe

Solids, i.e. three-dimensional shapes, also have a place in sacred geometry. The most commonly-mentioned solids in sacred geometry are the so-called Platonic solids . Although Plato did not create these solids himself, they are named after him, as he mentions the term in his dialogue Timaeus. In this work, the Greek philosopher discusses five different types of solids, which are the tetrahedron, hexahedron (or cube), octahedron, dodecahedron, and icosahedron, which have four, six, eight, twelve, and twenty faces respectively.

Plato associates his solids with the four basic elements. The tetrahedron, for instance, represented fire, due to its sharp points and edge, whereas the hexahedron was linked with earth, due to its four-square regularity. The octahedron and icosahedron, both of which are composed of triangles, represent air and water respectively. Lastly, the dodecahedron was assigned by Plato to the heavens, as its twelve pentagonal faces corresponded with the twelve constellations. Thus, using these five solids, Plato created a theory of the universe.

The Platonic solids are also found in Euclid’s Elements, though, unlike Plato, the mathematician seems to have been less interested in their symbolic properties. Instead, Euclid, in the last book of his Elements, describes the solids mathematically, and proves that exactly five regular solids exist. Plato’s use of the five solids to explain the universe was more or less discarded in the centuries that followed, and was only revived during the 16th century by the German astronomer Johannes Kepler . Since then, the Platonic solids have made their way into sacred geometry, and acquired additional symbolic value. For instance, the solids have been associated with the Hindu concept of chakra, the nodes of psychic energy in the body. The hexahedron, for instance, has been linked with the root chakra, the octahedron with the heart chakra, and the icosahedron with the sacral chakra.

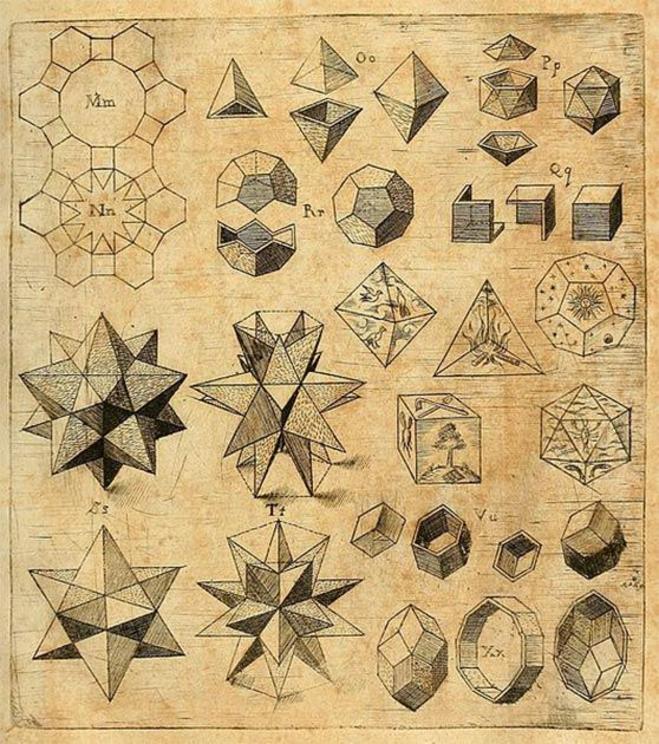Platonic solids were revived during the 16 th century by German astronomer Jogannes Kepler, in his Harmonices Mundi.

To conclude, sacred geometry has been an important means of explaining the world around us. Sacred geometry has been employed by various cultures throughout history, and continues to be applied in the modern era. Supporters of sacred geometry believe that this branch of mathematics holds the key to unlocking the secrets of the universe. Conversely, critics have argued that the data may be tailored to fit the theories. In other words, it is possible for proponents of sacred geometry to apply their beliefs onto anything.